Ideal gas for small pressure deviations

A special case of a linear elastic isotropic material is an ideal gas for small pressure deviations. From the ideal gas equation one finds that the pressure deviation $dp$ is related to a density change $d \rho$ by

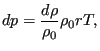$$dp=\frac{d \rho}{\rho_0} \rho_0 r T,$$ (374)

where $\rho_0$ is the density at rest, $r$ is the specific gas constant and $T$ is the temperature in Kelvin. Since $\rho V = \rho_0 V_0$ one obtains at $\rho=\rho_0$ and $V=V_0$:

$$V_0 d \rho + \rho_0 dV = 0$$ (375)

from which

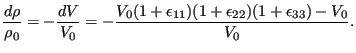$$\frac{d \rho }{\rho_0} = - \frac{dV}{V_0} = -\frac{V_0(1+\epsilon_{11})(1+\epsilon_{22})(1+\epsilon_{33})- V_0 }{V_0}.$$ (376)

From this one can derive the equations

$$-dp = dt_{11}=dt_{22}=dt_{33}=(\epsilon_{11}+\epsilon_{22}+\epsilon_{33}) \rho_0 r T$$ (377)

and

$$dt_{12}=dt_{13}=dt_{23}=0,$$ (378)

where $\boldsymbol{t}$ denotes the stress and $\boldsymbol{\epsilon}$ the linear strain. This means that an ideal gas can be modeled as an isotropic elastic material with Lamé constants $\lambda=\rho_0 r T$ and $\mu=0$. This corresponds to a Young's modulus $E=0$ and a Poisson coefficient $\nu=0.5$. Since the latter values lead to numerical difficulties it is advantageous to define the ideal gas as an orthotropic material with $D_{1111}=D_{2222}=D_{3333}=D_{1122}=D_{1133}=D_{2233}=\lambda$ and $D_{1212}=D_{1313}=D_{2323}=0$.